Notes on Algebraic Number Theory

Algebraic Number Theory

最近事情比较多，代数数论先断更一段时间，搞完毕设再来续。—— 2022 3.1

Introduction

primary concept

free abelian group

G is an abelian group with a basis, then any element in G could be uniquely expressed as an integer combination of finitely basis elements, like a 2-dim lattice in $\mathbb{Z}$.

module

module is an algebraic structure which is extension of vector space, scalar multiplication of module could be a ring instead of a field in vector space.

ideal is a kind of module, more details could be seen here.

field of fractions

Frac(R) means a field is defined based on a integral domain R like Q based on Z, which uses an equivalence relation on $R\times R\backslash \{0\}$ by letting $(n, d) \sim (m, b)$ whenever $nb =md$.

fractional ideal

A fractional ideal $I$ of R is an R-submodule, and it’s a subgroup of Frac(R). There exists a non-zero $r \in R$ let $rI \subset R$.

proper ideal

Any ideal of a ring which is strictly smaller than the whole ring.

principal ideal

An ideal which is generated by a single element, like $(2) \subset \mathbb{Z}$.

exact sequence

A sequence of group homomorphisms between groups like:

$G_0 \stackrel{f_1}\rightarrow G_1 \stackrel{f_2}\rightarrow \dots \stackrel{f_n}\rightarrow G_n$, $im(f_i) = ker(f_{i+1})$

$0$ or $1$ in sequence always mean trivial group $\{0\}, \{1\}$.

for sequences whose form like $0 \rightarrow A \stackrel{f}\rightarrow B \stackrel{g}\rightarrow C \rightarrow 0$, which is called ‘short exact sequences’. $f$ is monomorphism (injective or one-to-one) and $g$ is an epimorphism

symbol

$K^{\times}$: the multiplicative group of units in K.

$O_K$ : the ring of integers in field K, which contains all algebraic integers in K.

p8

The definition of norm map is in Field norm, $N_{L/K} (\alpha) = \prod_{\sigma\in Gal(L/K)} \sigma(\alpha)$

this is multiplicative, the details of norm could be found in p31

p10

There’s two errors here

$(1+\sqrt{-5})^2 = -4 + 2\sqrt{-5}$, but $(2, 1 + \sqrt{-5})^2$ still be equal to $(2)$.
$(2, 1+\sqrt{-5})(3, 1-\sqrt{-5})$ should be $(6, 3+3\sqrt{-5}, 2-2\sqrt{-5})$
1. as book says, $(2, 1+\sqrt{-5})^2 = (4, -4 + 2\sqrt{-5}, 2+2\sqrt{-5}) = (2)$
2. $(3, 1+\sqrt{-5})(3, 1-\sqrt{-5}) = (9, 3+3\sqrt{-5}, 3-3\sqrt{-5}, 6) \subset (3)$
  
  $3 = 9-6 \in (9, 3+3\sqrt{-5}, 3-3\sqrt{-5}, 6)$
  
  so $(3, 1+\sqrt{-5})(3, 1-\sqrt{-5}) = (3)$
3. $(2, 1+\sqrt{-5})(3, 1+\sqrt{-5}) = (6, 3+3\sqrt{-5}, 2+2\sqrt{-5}, -4 + 2\sqrt{-5})$
  
  $6 = (1+\sqrt{-5})(1-\sqrt{-5})$, $-4 + 2\sqrt{-5}= (1+\sqrt{-5})^2$
  
  $(2, 1+\sqrt{-5})(3, 1+\sqrt{-5}) = (1+\sqrt{-5})$
  
  so $(2, 1+\sqrt{-5})(3, 1+\sqrt{-5}) \subset (1+\sqrt{-5})$
  
  and $1+ =3+3 - (2+2) (2, 1+)(3, 1+) $
  
  so $(2, 1+\sqrt{-5})(3, 1+\sqrt{-5}) = (1+\sqrt{-5})$
4. $(2, 1+\sqrt{-5})(3, 1-\sqrt{-5}) = (6, 3+3\sqrt{-5}, 2-2\sqrt{-5})$
  
  $1-\sqrt{-5} = 6 - (3+3\sqrt{-5} + 2-2\sqrt{-5}) \in (6, 3+3\sqrt{-5}, 2-2\sqrt{-5})$
  
  $(6, 3+3\sqrt{-5}, 2-2\sqrt{-5}) \subset (1+\sqrt{-5}, 1-\sqrt{-5})$

p11

doubtful point:

units of $\mathbb{Z}[\sqrt2]$ are not only $1+\sqrt 2$, why $\mathbb{Z}[\sqrt 2]^{\times} = \{\pm(1+\sqrt 2)^m | m \in \mathbb{Z}\}$?

maybe in $\mathbb{Z}[\sqrt 2]^{\times}$, we could still use addition?

exercises

$d$, a square-free number, $K = \mathbb{Q}[\sqrt d]$, we talk about $O_K$ here.

Let $\alpha = a+b \sqrt d, b \neq 0, a, b\in \mathbb{Q}$, then if $\alpha \in O_K$,

it should satisfy $2a \in \mathbb{Z}, a^2-b^2d\in \mathbb{Z}$

If $a, b \in \mathbb{Z}$, it’s obviously that the condition are met whatever d is.

if $a \notin \mathbb{Z}$, it should be a half-integer, set $a = \frac{1}{2} + a'$, $\frac{1-4b^2d}{4}$ should be an integer, which is equivalent to $4b^2d \equiv 1 \mod 4$. if this work , the coefficient $4$ should be cancelled, and $4b^2d$ should be an integer, so b is a half-integer too, set $b = b' + \frac{1}{2}$, then it turns to $d \equiv 1\mod 4$.

After all, if $d \equiv 1 \mod 4$, $a, b$ could be all half-integer or all integer, else $a, b$ should be all integer.
In $\mathbb{Z}[\sqrt{-5}]$, proof $(6) = (2, 1+\sqrt{-5})^2(3, 1+\sqrt{-5})(3, 1-\sqrt{-5})$ is a factorization of $(6)$ into a product of prime ideals.
1. $(2, 1+\sqrt{-5})$ is prime means:
  
  $\forall a, b\in \mathbb{Z},$ if $(2a+(1+\sqrt{-5})b)\ |\ (cd),\ c, d \in \mathbb{Z}[\sqrt{-5}]$
  
  then $c \in (2, 1+\sqrt{-5})$ or $d \in (2, 1+\sqrt{-5})$.
  
  Let $c = c_0 + c_1\sqrt{-5}, d = d_0 + d_1\sqrt{-5}$, then we have
  
  $2(2a^2 + 3b^2+2ab)\ |\ (c_0^2+5c_1^2)(d_0^2+5d_1^2)$
  
  $2$ is prime, which means $(c_0^2 + 5c_1^2) \in (2)$ or $(d_0^2 + 5d_1^2) \in (2)$
  
  so $(2, 1+\sqrt{-5})$ is prime.
2. $(3, 1+\sqrt{-5})$ is prime means:
  
  $\forall a, b\in \mathbb{Z},$ if $(3a+(1+\sqrt{-5})b)\ |\ (cd),\ c, d \in \mathbb{Z}[\sqrt{-5}]$
  
  then $c \in (3, 1+\sqrt{-5})$ or $d \in (3, 1+\sqrt{-5})$.
  
  Let $c = c_0 + c_1\sqrt{-5}, d = d_0 + d_1\sqrt{-5}$, then we have
  
  $3(3a^2 + 2b^2+2ab)\ | \ (c_0^2+5c_1^2)(d_0^2+5d_1^2)$
  
  $3$ is prime, which means $(c_0^2 + 5c_1^2) \in (3)$ or $(d_0^2 + 5d_1^2) \in (3))$
  
  so $(3, 1+\sqrt{-5})$ is prime.
3. In a similar way, we could also prove that $(3, 1-\sqrt{-5})$ is prime.

Commutative Algebra

some details in this chapter would be found here.

primary concept

quotient ring

use an equivalence relation $\sim$ on a ring $R$, $I$ is a two-sided ideal in R:

$a b $ if and only if $(a-b) \in I$

$a \mod I$ is the equivalence class of element $a \in R$, $[a] = a+I:=\{a+r|r\in I\}$

we call $R/I$ a quotient ring which is $\{a \mod I | a\in R\}$

zero-element in $R/I$ is $I$, multiplicateive identity is $1+I$

Euclidean domain

A Euclidean domain is an integral domain which can be endowed with at least one Euclidean function.

A Euclidean function on an integral domain R is a function $f: R\backslash\{0\} \rightarrow \{a\ | \ a \geq 0\}$ which satisfies:

$\forall a, b \in R, b \neq 0$, there exist $q, r \in R,\quad a = b q + r$

and $r= 0$ or $f(r) < f(b)$

algebra

Let $K$ be a field, and let $A$ be a vector space over K, if there’s a bilinear map $A \times A \rightarrow A$ on A, then we call $A$ as $K$-algebra, and $K$ is the base field of $A$.

symbol

\[{\rm Hom}\]: For two modules $M, N$ over a ring $R$, ${\rm Hom}_R(M, N)$ denotes the set of all module homomorphisms from $M$ to $N$.

p17

In the first one of Nakayama’s Lemma, $\mathfrak{a}$ should be an ideal contained in all maximal ideals of $A$, this make $1-a_1$ not be in $\mathfrak{m}$, more details could be seen here.

p18

$\mathfrak{a}^e \in S^{-1}A$ means $\{a/s \ | \ a \in \mathfrak{a}, s\in S\}$

For $\mathfrak{a}$ an a ideal of $S^{-1}A$, $\mathfrak{a} \cap A$ means all integer in $\mathfrak{a}$.

p19

'$\mathfrak{p}$ a prime ideal in $S^{-1}A \Rightarrow \mathfrak{p} \cap A$ is a prime ideal in $A$ disjoint from $S$’ is not clear, $\mathfrak{p} = \{\frac{a}{b} \ | \ a\in \mathfrak{p}', b \in S\}$ has to satisfies $\mathfrak{p}' \cap S = \varnothing$

$A_{\mathfrak{p}}$ means $(A-\mathfrak{p})^{-1}A$, more details could be seen here.

Conversely thinking, if there’s another genertors $w$ in $\mathfrak{p}A_{\mathfrak{p}}$, then $w (A-)^{-1}(A-) $, it would make $\mathfrak{p}A_{\mathfrak{p}}$ and $A$ equal. We could use some examples in $\mathbb{Z}$ to help us understand.

To understand $(m) + (n) = \mathbb{Z}$ if and only if $m$ and $n$ are relatively prime. We could think back to $GCD$ on $\mathbb{Z}$, if $GCD(m, n) = 1$, then $\exists\ s, t, \ sm+tn = 1$.

p20

$\mathfrak{a} \mathfrak{b} = \{\sum_ia_ib_i\ | \ a_i\in \mathfrak{a}, b_i\in \mathfrak{b}\}, c\in \mathfrak{a}_1 \cap \mathfrak{a}_2$, which means $a_1c+a_2c \in \mathfrak{a_1} \mathfrak{a_2}$.

the detailed proof of $A/(\mathfrak{a}_1 \cap \mathfrak{a}_2) \simeq A/\mathfrak{a}_1 \times A/ \mathfrak{a}_2$ could be seen here.

p21

doubtful point: element in $\otimes_iM_i$ should be like $(m_0, m_1, \dots)$, but why the isomorphism map be $(\sum m_i) \otimes (\sum n_j) \rightarrow \sum m_i \otimes n_j$

p22

proof for $M/aM (A/)_A M $ could be seen here. The point is why $im(\mathfrak{a} \otimes_A M) \cap M = \mathfrak{a}M = \{\sum a_im_i \ | \ a_i \in \mathfrak{a}, m_i \in M\}$, firstly I think for different bilinear map $f$, then $\mathfrak{a} \otimes_A M$ should be different. But then I realized that all the rings are isomorphic, so we could use an simple bilinear map $f(a, b) = a \cdot b$, then we get $im(\mathfrak{a} \otimes_A M) \cap M= \{\sum a_im_i \ | \ a_i \in \mathfrak{a}, m_i \in M\}$.

For counter-example about $M \rightarrow N$ is injective but $M \otimes_A P \rightarrow N \otimes_A P$ is not.

We know that $M \otimes_A P \rightarrow N \otimes_A P = (M\rightarrow N)\otimes_AP$

Let $A = \mathbb{Z}, M = \mathbb{Z}, N = m\mathbb{Z}, P = \mathbb{Z}/ m\mathbb{Z}$, then $M \rightarrow N$ s injective, and $M \otimes_A P \rightarrow N \otimes_A P$ is a zero map.

p23

the reason of using $m \rightarrow 1\otimes m$ to denote the map from $M$ to $B \otimes_A M$ is that $B\otimes_A M \simeq \{bm\ | \ b\in B, m\in M \} \simeq \{1 \otimes m\ | \ m \in M\}$

Because I’m not familiar with field, so maybe compelete notes on this part later.

exercises

If $S$ is saturated, we assumpt that its complement isnot a union of prime ideals, which means there’s at least one prime ideal $I$ satisfies:

$\exists I_0 \subseteq S,\ I_0 \not \subset A\backslash S,\quad \ I\backslash I_0 \subseteq A\backslash S,\ I\backslash I_0\not \subset S$

then because of the property of being saturated , $\exists i_0 \in I_0, i_0 \in S$, $i_0$ is prime, so $\{1, i_0, i_0^2, \dots, i_0^n, \dots \} \subseteq S$. Let $U$ be the set of all the units, because $1 \in S$, then $U \subseteq S$. For all the prime ideals with one generator $(i_0), (i_1), , $ the set $\{1, i_j, i_j^2, \dots, i_j^n, \dots \} = A\backslash U - \cup_{ k\neq j}(i_k)$.

so in this case, all the elements in $I\backslash I_0$ could still be allocated to other prime ideals, the assumption is false.

If complement of $S$ is a union of prime ideals, then according to above proof, S should be like $A- \cup_{ k\notin J}(i_k) = \cup_{j\in J}\{1, i_j, i_j^2, \dots,i_j^n,\dots \} \cup U$, then $\forall s \in S$, the form of it is $\prod_{j\in J} i_j^{e_j}, e_j \in \mathbb{Z}$, clearly it’s saturated.
According to 1, we know that $S' = S$ could satisfy the need.

For $S^{-1}A = \{\frac{a}{s} \ | \ a\in A, s \in S\}$, the form of $\frac{a}{s}$ is $\frac{a}{\prod_{j\in J} i_j^{e_j}} , e_j \in \mathbb{Z}$.

so for prime ideal $i_w \in A$, $(\frac{1}{i_w})$ is still a prime ideal in $S^{-1}A$.

Rings of Integers

primary concept

separable extension

for an algebraic field enxtension $E \supseteq F$ is called a separable extension if $\forall \alpha \in E$, its minimal polybomial over $F$ is a separable polynomial (the polynomial’s roots are distinct, without multiple root).

dual space

For a vector space $V$ over a field $F$, dual space $V^{\lor}$ is the set of all linear maps from $V$ to $F$.

Kronecker delta

$\delta_{ij} = 0$ if $i \neq j$ else $1$.

stem field

$K$ a field, and $f \in K[x]$ is an irreducible polynomial. The ring $L = K[x]/(f)$ is a field, with a distinguished root $\overline x = x + (f)$ of $f$. The stem field of $f$ is the pair $(L, \overline x)$.

symbol

$(k_{ij})$: sometime means a matrix $M$ satisfy $M_{ij} = k_{ij}$

p25

symmetric polynomial means all the coefficients in the polynomial has equal status.

p26

Cause $h(X)$ is a product of monomials, so we could take $g(\alpha_{\sigma(1)}, \dots,\alpha_{\sigma(n))})$ as $g_i(\alpha_1, \dots, \alpha_n)$, then the coefficient polynomials would be symmetric after expanding the product.

The point is: for any integral elements $ , $, $\alpha +\beta, \alpha \beta$ are still integral elements.

For the first proof, the chain of thought should be:

‘symmetric polynomials are polynomials of symmetric elementary polynomials’ $\Rightarrow$

‘plug integral elements in any polynomial in $A[X]$ would get other integral elements.’ $\Rightarrow$

then we use polynomials $x+y / xy$ to get ‘all integral elements form a ring’.

At the end of the second proof, the point is $\alpha \beta M, (\alpha \beta) M$ all in $M$.

p28

another proof of Proposition 2.9 could be seen here. $a, b$ are not random elements in $A$.

p29

For proposition 2.11, firstly I’m confused about ‘some $a_i \in A$’, if $$ is integral over A, then all $a_i$ should be in $A$. The rest part of proof are clearly, we know all integral elements form a ring, so if all the roots are integral over $A$, and so are all the coefficients. The proof in book has proved that if an element $\alpha$ of $L$ is integral over $A$, then all the coefficients of its minimal polynomial over K are in $A$.

And conversely, I know ‘some’ works that if there’s no coefficient of $\alpha$ minimal polynomial over $K$ is in $A$, then $\alpha$ would not be integral over $A$.

Also, I’m confused about proposition 2.13, any A-algebra should be a vector space, then it should be a A-module, why need to prove that.

p31

There’s an example could help us to understand the determinant of a basis of module works here.

The proof of ‘index of $N$ is $det(a_{ij})$’ could be seen here. We could associate it with the relation with determinant of lattice and sublattice (In $\mathbb{Z}^n$, determinant of sublattice is multiple of determinant of lattice.).

trace and norm

Consider $L/K$ as an extension of fields of degree $n$. The trace of $x \in L$ is the trace of the $K$-linear endomorphism $m_x: L\rightarrow L, m_x(y) = xy$, the norm of $x$ is $det(m_x)$.

Computational procedure (for element $ x L$, basis is $\{e_0,\dots, e_{n-1}\}$):

calculate vector: $\vec m_{xy} = x (e_0y_0, \dots, e_{n-1}y_{n-1})$

calculate matrix: $m_x : m_x \times \vec m_{xy} = (y_0, \dots, y_{n-1})$

calculate trace and norm: $Tr(x) = Tr(m_x), Nm(x) = det(m_x)$

For proposition 2.19, it build a field $K[\beta]$ in the middle to complete the proof. $L = K[\beta]$ in the proof is not the previous $L$. For $L/K$ with basis $\{1, \beta, \beta^2, \dots, \beta^{n-1}\}$, we could get trace and norm as follow $$ f(x) = x^n - 1x^{n-1} - - {n-1}x - _n \

= _1, f(_i) = 0, i = 1, 2, , n-1\

m_x = \[\begin{bmatrix} 0 &\cdots & 0 & \alpha_n\\ 1 & \cdots & 0 & \alpha_{n-1}\\ \vdots & \ddots & \vdots & \vdots\\ 0 & \cdots & 1 & \alpha_1 \end{bmatrix}\]

$$ use Vieta’s formulas, we get $Tr(\beta) = \alpha_{1} = \sum \beta_i, Nm(\beta) =(-1)^n\alpha_n = \prod \beta_i$, and 2.19 extend it to some $L$ with degree bigger than $n$.

p32

In corollary 2.20, $\sigma_i$ are all homomorphisms, $\sigma_i \beta$ means $\sigma_i(\beta)$

In this page, I’m confused the difference between module and extension, because in $Tr_{B/A}$, somtimes $B$ is an extension over $A$, sometimes $B$ is $A$-module. The point is that we could think that if $B$ is a $A$-module which is a 2-dims vector space, then we couldn’t define the polynomial for $B/A$, how could we calculate $Tr_{B/A}$.

Then we could see here, the second answer gives a construction for vector space like module, then we know the $Tr$ is just an operator, if meet its property, it could exist, in general case, it’s called the Hattori-Stallings trace.

p33

How to construct the isomorphism from $V^{\lor}$ to $V$ could be seen here.

If got any more question about discriminant, you may get the answer here.

p35

For corollary 2.30, I think we could know the basis $\{\beta_1, \dots, \beta_m\}$ of $L$ could all in $O_L$, so the subring of $L$ which is finitely generated as a $\mathbb{Z}$-module is also generated by basis in $O_L$, clearly $O_L$ is the largest subring.

p36

In Remark 2.33, $Tr(\beta \beta_i) \in A , i=1, \dots, m$ means that a ‘dual’ basis $\{\beta_1', \dots, \beta_m'\}$ is in $C^*$, then we know the conclusion from 2.29.

p39

If $D(1, \alpha, \dots, \alpha^{m-1})$ doesn’t contain any square factor, then if should be $disc(O_K/\mathbb{Z})$, and $|O_K| = |\mathbb{Z}[\alpha]|$, then we knwo $O_K = \mathbb{Z}[\alpha]$.

sign function here just means the plus or minus of integer.

According to 2.34, we know $D(1, \beta, \dots, \beta^{m-1}) = \prod_{1\leq i\leq j\leq m}(\beta_i-\beta_j)^2$, then we know 2.40(a).

detailed proof of Stickllberger’s Theorem could be seen here.

proof for $a^2 , 1, a $

if $\exists a\in \mathbb{Z}, a^2 \equiv 2 \mod 4$, then $a^2 = 4k+2 = 2(2k+1)$, the right side only has one 2 factor, so there’s a contradiction.

if $\exists a \in \mathbb{Z}, a\equiv 3\mod 4$, then $a^2-1 = 4k+2 \Rightarrow (a+1)(a-1)=2(2k+1)$, the left side has two 2 factor, but the right side only has one.

p41

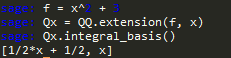
I use sagemath to do the same work as PARI do in the book.

exercise

To find an example, we want $\alpha = (a+b\sqrt 5)(c+d\sqrt 5) = (e+f\sqrt 5)(g + h\sqrt 5)$ with $a \neq e, a \neq g$, so the variables should satisfy $ac + 5bd = eg + 5hf, ad + bc = eh+ fg$.

While, it’s too bother, let’s just use $-4 = -2 \cdot 2 = (1+\sqrt 5)\cdot (1-\sqrt 5)$.
If $f(X)$ is reducible in $K[X]$, which means $f(X) = f_0(X)f_1(X)...fn(X)$ with $f_i(X) \in K[X]$. We know that $f(X) \in A[X]$, so all the roots of $f(X)$ are integral over $A$. For $f_0(X)$, the roots of $f_0$ are all integral over A, so all the coefficients should be integral over $A$, then they are all in $A$.
Let $L = K[\beta]$, $\beta$ is one root of $f(X) \in K[X]$. From 2.34, we know that $disc(L/K) = (-1)^{\frac{m(m-1)}{2}}\prod_i(\prod_{j\neq i} (\beta_i-\beta_j))$, $\beta_1=\beta, \beta_2, \dots, \beta_n$ are the roots of $f(X)$, because $L/K$ is not separable, then there exists $\beta_s =\beta_t$, $disc(L/K) = 0$.
$\mathfrak{a} \neq (2)$ is clearly, $\mathfrak{a}^2 = (4, 2+2\sqrt{-3}, -2+2\sqrt{-3})$, $-2+2\sqrt{-3} = 2+2\sqrt{-3} - 4$, then $\mathfrak{a}^2 = (4, 2+2\sqrt{-3}) = (2)\mathfrak{a}$.

In $\mathbb{Z}[\sqrt{-3}]$, there exists $10 = 2 \cdot 5 = (1+\sqrt{-3})(1-\sqrt{-3})$, so it couldn’t factor uniquely.
For $\alpha \in A[\beta] \cap A[\beta ^{-1}]$, there will be two polynomials $f(X) \in A[\beta][X]$, $g(X)\in A[\beta^{-1}][X]$, with $f() = g() = $, and the coefficients of two polynomials are all in $A$, we let $deg(f) = m, deg(g) = n$

We set $M = A + A\beta + \dots + A\beta^{m+n}$, then we wonder if $\alpha \beta^k$ is in $M$.
1. $0 \leq k < n$: $\alpha \beta^k = \beta^kf(\beta) \in M$
2. $n\leq k \leq m+n$: $\alpha \beta^k = \beta^{k-n} \alpha \beta^{n} \in M$
then we know that $\alpha \beta^k \in M$, so $\alpha M \in M$, then $$ is integral over $A$, more details could be seen here.
1. All $\alpha_i \alpha_j, i\neq j$ would have factor $(1+\sqrt{10})(1-\sqrt{10}) = -9$ or $(1+\sqrt{7})(1-\sqrt{7}) = -6$, they are all divisible by 3 in $O_K$. According to 2.12, we know that if $\frac{\alpha_i^n}{3}$ is integral over $\mathbb{Z}$, then its norm and trace should all in $\mathbb{Z}$, the norm clearly lie in $\mathbb{Z}$, but the trace is not. We know $Tr(\alpha_i^n) = \sum \alpha_j^n = 4^n$, because for $f(X) \in \mathbb{Z}[X], f(\alpha_j) = 0$, then the minimal polynomial $f_n(X)$ of $\alpha_i^n$ would satisfy $f_n(\alpha_j^n) = 0$.
2. If $\overline g$ is divisible by $f$ in $F_3[X]$, then $\overline g(\alpha) = 0$ over $F_3$, and $g(X) = \overline g(X) + 3g'(X)$, so $g(\alpha) \equiv \overline g(\alpha) \mod 3$.
  
  if $g(\alpha)$ is divisible by 3 in $\mathbb{Z}[\alpha]$, then $\overline g(\alpha) = 0$ in $F_3$, because $f$ is minimal polynomial of $\alpha$, then $\overline g$ is divisible by $f$. The point is that whether $f$ is still minimal polynomial in $F_3[X]$. If there’s another $f' \in F_3[X], f'(\alpha) = 0$ with $deg(f') < deg(f)$, then $f'(\alpha) + 3T = 0$ over $\mathbb{Z}$, $f''(X) = f'(X) + 3T$ become minimal polynomial, so there’s a contradicition.
3. We know that $f_i(\alpha) \equiv \overline f_i(\alpha) \mod 3$, then $\overline f_i \overline f_j(\alpha) \equiv \alpha_i\alpha_j \mod 3$, from 1 we know $\alpha_i\alpha_j \equiv 0\mod 3$, so $\overline f | \overline f_i \overline f_j, i\neq j$, and $\overline f$ not divide $\overline f_i^n$, because if it happens, then $\overline f_i^n(\alpha) = 0$, which means $\alpha_i^n \equiv 0 \mod 3$, which is a contradiction.
4. $\overline f$ has at least 4 irreducible factors on $F_3[X]$, which means $f$ has at least 4 irreducible factors on $\mathbb{Z}[X]$, and $f$ has degree at most 4, so $f$ just has these 4 irreducible factors, $f$ will be $f(X) = (X-t_0)(X-t_1)(X-t_2)(X-t_3)$, we know $f(\alpha) = 0$, so $t_i = \alpha$, then $X-t_i$ is the minipoly of $\alpha$, here’s a contradiction.
$S^{-1}A = \{\frac{a}{s}\ |\ a\in A, s\in S \}$, $\forall f(X) \in S^{-1}A[X]$, $f(X)$ could be written as $\frac{\sum_{i=0}^na_i'X^i}{\prod_{i=0}^ns_i}$, if $f(x_0) = 0$, then there exists $f'(X)$ with $f'(x_0) = 0$ on $A$, so $S^{-1}B$ is still integral closure of $S^{-1}A$.
$A_\mathfrak{p} = \{\frac{a}{s}\ | \ a\in A, s\in A\backslash \mathfrak{p}\}$, and $\mathfrak{p}A_\mathfrak{p} = (A \backslash \mathfrak{p}) ^{-1} \mathfrak{p} = \{\frac{p}{s} \ | \ p\in \mathfrak{p}, s \in A\backslash \mathfrak{p}\}$.

$A_\mathfrak{p}/ \mathfrak{p}A_\mathfrak{p} = \{\frac{a}{s} \ | \ a\in A/\mathfrak{p}A, s\in A/\mathfrak{p} \}$，then $\forall p \in A_\mathfrak{p}/\mathfrak{p}A_\mathfrak{p}$ can be written in the form $p = \frac{a}{b}, a, b\in A$.

Dedekind Domains & Factorization

We could know that the first main task of this book is to summarize what a kind of ring could have the property which is factorable uniquely.

p49

In alternative proof, there’s an error that $a \in \mathfrak{a}, b\in \mathfrak{b}$, not $A$ and $B$.

In lemma 3.10, the map is $a + \mathfrak{p}^m \rightarrow a + \mathfrak{q}^m$, firstly I thought it should be a map $A/\mathfrak{p}^m \rightarrow A/\mathfrak{q}^m$, but then I realized that $\mathfrak{q}^m$ is not ideal of $A$, so this quotient ring couldn’t exist. Then I take a look at the equivalence, we want to prove the map is one-to-one, which equals $\forall a_0, a_1\in A$, if $a_0 + \mathfrak{q}^m = a_1+\mathfrak{q}^m$, then $a_0+\mathfrak{p}^m=a_1 + \mathfrak{p}^m$. If $a_0 = a_1$, it’s obvious, if not, then $a_0 \neq a_1, a_0+\mathfrak{q}^m = a_1 + \mathfrak{q}^m$, hence $a_0, a_1 \in A\cap \mathfrak{q}^m$, so in the proof we have to show that $\mathfrak{q}^m \cap A = \mathfrak{p}^m$.

p50

Firstly I’m confused about $s^{-1}$ in $A_\mathfrak{p}/\mathfrak{q}^m$, the element in $A_\mathfrak{p}/\mathfrak{q}^m$ should be in the form of $a + \mathfrak{q}^m$, $s$ is just an element in $A\backslash \mathfrak{p}$, so $s^{-1}$ is also a set $\{b \ | \ bs + q = 1, q \in \mathfrak{p}^m\}$. $ba = \frac{a}{s}(1-q)$, then $\frac{a}{s}(1-q) + \mathfrak{q}^m = \frac{a}{s} + \mathfrak{q}^m$, then I know that “invertible” means $b + \mathfrak{q}^m$ is the inverse of $s + \mathfrak{q}^m$.

In the proof, firstly we need to know the isomorphism from the ideals of $A/\mathfrak{b}$ to the ideals of $A$ containing $\mathfrak{b}$, details could be seen here.

For $A/ , $ $\mathfrak{q}_i^j = (A\backslash \mathfrak{p}_i)^{-1}\mathfrak{p}^j$ , and for $\mathfrak{a}/\mathfrak{b}$, here we take $\mathfrak{a}/\mathfrak{b}$ as an element in $A/\mathfrak{b}$, then we know that $\mathfrak{p}_1^{s_1}\mathfrak{p}_2^{s_2}\dots\mathfrak{p}_m^{s_m} / \mathfrak{b}$ is also an element in $A/\mathfrak{b}$, we take this element in the isomorphism, under first isomorphism, it turns to $\mathfrak{p}_1^{s_1}\mathfrak{p}_2^{s_2}\dots\mathfrak{p}_m^{s_m} / \mathfrak{p}_1^{r_1} \times \dots \times \mathfrak{p}_1^{s_1}\mathfrak{p}_2^{s_2}\dots\mathfrak{p}_m^{s_m} / \mathfrak{p}_m^{r_m}$, we could use an example $(2 ) / (4) $ over $\mathbb{Z}$ to think of $\mathfrak{p}_1^{s_1}\mathfrak{p}_2^{s_2}\dots\mathfrak{p}_m^{s_m} / \mathfrak{p}_1^{r_1}$, then we know that $\mathfrak{p}_1^{s_1}\mathfrak{p}_2^{s_2}\dots\mathfrak{p}_m^{s_m} / \mathfrak{p}_1^{r_1} \cong \mathfrak{p}_1^{s_1} / \mathfrak{p}_1^{r_1}$, because the zero of both is $ {k _1^{r_1-s_1} | k }$. Then under the second isomorphism, $\mathfrak{p}^{s_i} \rightarrow \mathfrak{q}^{s_i} : A \rightarrow A_{\mathfrak{p}}$.

In the last, if $\mathfrak{q}_i^{s_i} = \mathfrak{q}_i^{t_i}$ with $s_i \neq t_i$, we suppose $s_i > t_i$ then there’s an ideal chain $\mathfrak{q}_i^{t_i} \subseteq \mathfrak{q}_i^{t_i-1} \subseteq \dots \subseteq \mathfrak{q}_i^{s_i} \subseteq \dots \subseteq \mathfrak{q}_i$, any ideal $ _i^{j}$ is in this chain, then $A_{ \mathfrak{p}_i}/ \mathfrak{q}_i$ is not a field, so $ _i$ is not the maximal ideal, which contradicts the premises.

p51

Firstly, I’m confused about $x\equiv 1 \mod \mathfrak{p}_i, i\neq 1$, how could we get this. Then I think the point is why $\mathfrak{p}_1$ and $(x)$ generate the same ideals in $A_{\mathfrak{p}_i}$. $\mathfrak{p}_1$ generates $\frac{\mathfrak{p}_1}{A-\mathfrak{p}_i}$, and $(x)$ generates $\frac{(x)}{A-\mathfrak{p}_i} = \frac{(x_1) + \mathfrak{p}_1^2}{A-\mathfrak{p}_i} = \frac{k_1\mathfrak{p}_1 + \mathfrak{p}_1^2}{A-\mathfrak{p}_i} = \frac{\mathfrak{p}_1(k_1 + \mathfrak{p}_1)}{A-\mathfrak{p}_i}$, we know that $x_1 \in \mathfrak{p}_1 - \mathfrak{p}_1^2$ , so $k_1 \notin \mathfrak{p}_1$, $k_1+\mathfrak{p}_1$ is fixed, then we have a simple isomorphism $\sigma(\frac{p}{q}) : \frac{p}{q} \rightarrow \frac{p}{(k_1+\mathfrak{p}_1)q}$ from $(x)$ to $\mathfrak{p}_1$.

3.14 and 3.15 use the same kind of proof, in 3.15, we could calculate that $\mathfrak{a}$ generates $\frac{\mathfrak{p}_i^{s_i}}{A-\mathfrak{p}_i}$, and $\mathfrak{b} + (a)$ generates $\frac{\mathfrak{p}_i^{r_i}}{A-\mathfrak{p}_i} + \frac{(x_i)}{A-\mathfrak{p}_i}$ in $A_{\mathfrak{p}_i}$, then I’m wondering whether $(a) = \mathfrak{a}$, but then I recognized that $a \equiv x_i \mod \mathfrak{p}_i^{r_i}$, so it need the part $\frac{\mathfrak{p}_i^{r_i}}{A-\mathfrak{p}_i}$.

p52

The point of proof for 3.18 is to use the (c) property of Dedekind domains.